Page 20 - RMGO 3

20 Thanos KALOGERAKIS

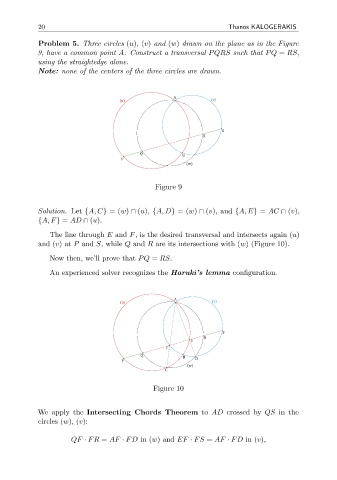
Problem 5. Three circles (u), (v) and (w) drawn on the plane as in the Figure
9, have a common point A. Construct a transversal PQRS such that PQ = RS,
using the straightedge alone.
Note: none of the centers of the three circles are drawn.

Figure 9

Solution. Let {A, C} = (w) ∩ (u), {A, D} = (w) ∩ (v), and {A, E} = AC ∩ (v),
{A, F} = AD ∩ (u).

The line through E and F, is the desired transversal and intersects again (u)
and (v) at P and S, while Q and R are its intersections with (w) (Figure 10).

Now then, we’ll prove that PQ = RS.

An experienced solver recognizes the Haruki’s lemma configuration.

Figure 10

We apply the Intersecting Chords Theorem to AD crossed by QS in the
circles (w), (v):

QF · FR = AF · FD in (w) and EF · FS = AF · FD in (v),