Page 17 - RMGO 3

Geometric constructions, with the compass alone, the straightedge alone or neither of them 17

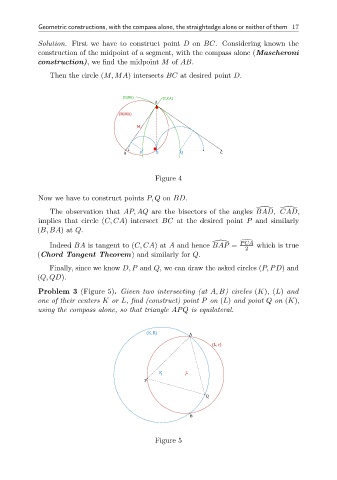
Solution. First we have to construct point D on BC. Considering known the
construction of the midpoint of a segment, with the compass alone (Mascheroni
construction), we find the midpoint M of AB.
Then the circle (M, MA) intersects BC at desired point D.

Figure 4

Now we have to construct points P, Q on BD.
The observation that AP, AQ are the bisectors of the angles BAD, CAD,
\ \
implies that circle (C, CA) intersect BC at the desired point P and similarly
(B, BA) at Q.
\
PCA
Indeed BA is tangent to (C, CA) at A and hence BAP = which is true
\
2
(Chord Tangent Theorem) and similarly for Q.
Finally, since we know D, P and Q, we can draw the asked circles (P, PD) and
(Q, QD).
Problem 3 (Figure 5). Given two intersecting (at A, B) circles (K), (L) and
one of their centers K or L, find (construct) point P on (L) and point Q on (K),
using the compass alone, so that triangle APQ is equilateral.

Figure 5