Page 15 - RMGO 3

ARTICOLE SI NOTE MATEMATICE
,

Geometric constructions, with the compass
alone, the straightedge alone or neither of them

Thanos KALOGERAKIS 1

Geometric constructions are, probably, some of the most exciting problems
among Euclidean geometry enthusiasts. Even more so, when they can be accom-
pliced using either the compass, or the straightedge alone.
Below we present three constructions, with the compass alone, three more with
the straightedge alone and one with neither of them.

Constructions with the compass alone

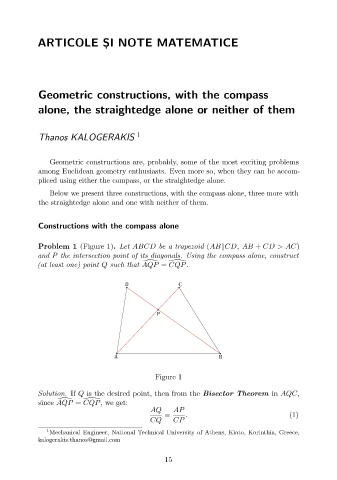
Problem 1 (Figure 1). Let ABCD be a trapezoid (ABkCD, AB + CD > AC)
and P the intersection point of its diagonals. Using the compass alone, construct
(at least one) point Q such that AQP = CQP.
[
\

Figure 1

Solution. If Q is the desired point, then from the Bisector Theorem in AQC,
\
since AQP = CQP, we get:
[
AQ AP
= . (1)
CQ CP
1
Mechanical Engineer, National Technical University of Athens, Kiato, Korinthia, Greece,
kalogerakis.thanos@gmail.com
15