Page 16 - RMGO 3

16 Thanos KALOGERAKIS

But, from the PDC, PAB triangles’ similarity, we have:
AB AP BP
= = . (2)
CD CP DP
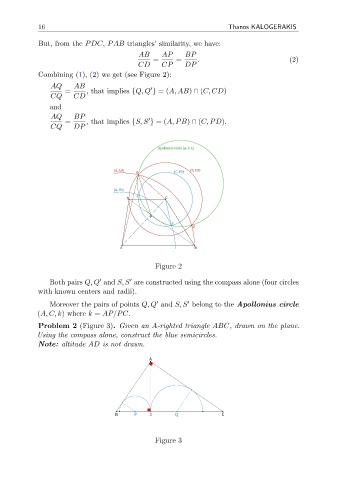
Combining (1), (2) we get (see Figure 2):
AQ AB
0
= , that implies {Q, Q } = (A, AB) ∩ (C, CD)
CQ CD
and
AQ BP
0
= , that implies {S, S } = (A, PB) ∩ (C, PD).
CQ DP

Figure 2
0
0
Both pairs Q, Q and S, S are constructed using the compass alone (four circles
with known centers and radii).
0
0
Moreover the pairs of points Q, Q and S, S belong to the Apollonius circle
(A, C, k) where k = AP/PC.
Problem 2 (Figure 3). Given an A-righted triangle ABC, drawn on the plane.
Using the compass alone, construct the blue semicircles.
Note: altitude AD is not drawn.

Figure 3