Page 18 - RMGO 3

18 Thanos KALOGERAKIS

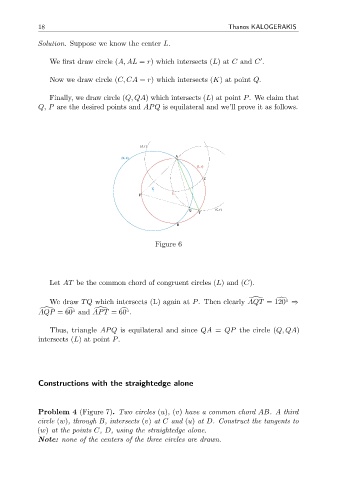
Solution. Suppose we know the center L.
0
We first draw circle (A, AL = r) which intersects (L) at C and C .

Now we draw circle (C, CA = r) which intersects (K) at point Q.

Finally, we draw circle (Q, QA) which intersects (L) at point P. We claim that
Q, P are the desired points and APQ is equilateral and we’ll prove it as follows.

Figure 6

Let AT be the common chord of congruent circles (L) and (C).

d ◦
We draw TQ which intersects (L) again at P. Then clearly AQT = 120 ⇒
[
c ◦
c ◦
[
[
AQP = 60 and APT = 60 .
Thus, triangle APQ is equilateral and since QA = QP the circle (Q, QA)
intersects (L) at point P.

Constructions with the straightedge alone

Problem 4 (Figure 7). Two circles (u), (v) have a common chord AB. A third
circle (w), through B, intersects (v) at C and (u) at D. Construct the tangents to
(w) at the points C, D, using the straightedge alone.
Note: none of the centers of the three circles are drawn.